3 CHANGING THE MOVEMENT OF AN OBJECT REQUIRES A NET FORCE TO BE ACTING ON IT

Task 10

Materials and tools for a working group:

2 drawing papers, sticking tape/glue, string, weight (eg plasticine)

It is good to recall that in the previous and the following task we operate with the concept of angle, while the pupils do not already conceptualize it. Anyway, it is not necessary to introduce this concept and in spite of that pupils are able to investigate the slanting throw. It is enough if we are discussing the pupils in terms of differentlyinclined shooting of a ball from a sling. Following this reason it is not appropriate to use real protractors, in the activity pupils construct their own measurement of the throw initiation. By creating a tool for measuring the inclination of the litter by the object, they will in principle understand what an angle is, even though we do not have to mislead the concept itself.

Pupils will construct a specific measurement tool. It is good to focus on the design of the tool itself and its use, so that the pupils understand what the meaning is in the following research. Therefore, the teacher, as soon as the research question is raised (How obliquely is it necessary to shoot the ball in order to reach it as far as possible?),discusses with the pupils how they can measure how the ball is obliquely thrown. Given the development of the science process, it is important to allow pupils in groups to try to design their own tools. If one of them is sufficiently objective, it is advisable to use the procedure proposed by the pupils.

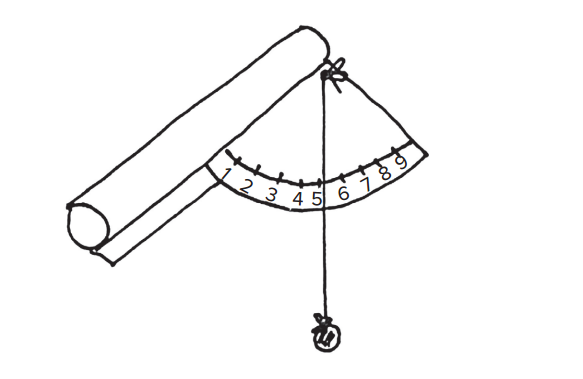
Pic 38: Instrument for measuring the angle of launch of objects

If pupils do not develop their own tool, the teacher can propose the creation of a simplified tool by himself (see Picture). First, they cut out a quarter circle from the drawing, to which the numbers from 1 to 8 (as if at 10 ° to 90 °, i.e. to the right angle; they do not need to be justified by the children) are given at regular intervals. Best if the teacher prepares these neighborhoods for pupils, it will better ensure regular stretches of measured angles. Alternatively, it can give pupils a template to draw the scale over. Then make a roll from the next drawing and glue it so that the edge of the roll protrudes. It will stick to it the produced „protrusion“. They make a hole in the corner of the protractor and hang a string with a weight, such as a piece of plasticine (see picture). The lanyard is a direction pointer. When the roll is tilted differently, the number on which the hanging cord shows is also changing. Pupils can look through a roll at a particular object and determine at what angle (as oblique) they will shoot if they target the object.

Pic 39: Object incidence chart

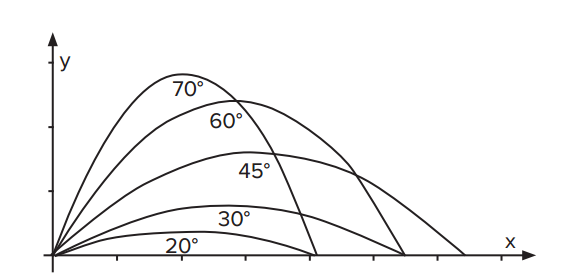
When pupils are familiar with how to use the constructed tool, they can approach the research question: How obliquely is it necessary to fire the ball to keep it flying as far as possible? However, for the development of science ideas, it is interesting to encourage pupils to try to verify that the diagram given in Task 10 expresses really where the objects will fall if we throw them in different directions. The task thus leads pupils to „read“ the information with the diagram. Although the data is given in degrees of angles, it is very easy to convert these degrees to numbers on their own scale (eg, 20 ° = 2; 30 ° = 3, 90 ° = 9, etc.).

Pupils can create their own table for measured data to carry out the research. It is not stated intentionally. In many of the previous tasks, pupils had examples of tables to systematize the researchdata, in this task they should try to create their own table, thereby better understanding the importance of creating tables. It is advisable to repeat each measurement multiple times, or to exchange data from multiple groups. On the basis of these data, they try to draw a conclusion to answer the research question. Either they answer the open question of whether it depends on where the object falls from how we shoot the ball sloping obliquely, or whether their measured data is in line with what the graph shows. For example, if the ball hit the same spot if it was fired from a „twos“ and „sevens“ on their own scale (a simplified twist). The basic conclusion is that the distance increases when we shoot an object from „unit“ to „four to five“, then the distance begins to reduce again.